Turbulence is a paradigm problem in non-equilibrium statistical physics, with systems exhibiting large fluctuations as well as a macroscopic space-time structure. Although the Navier-Stokes equations have been known for about 150 years, a general a priori methodology for calculating statistical averages of turbulent observables, such as drag forces or heat transfer rates, still does not exist.

Scientific Approach

Researchers will locate and characterize Unstable Periodic Orbits (UPOs) in weakly turbulent hydrodynamics described by the Navier-Stokes equations. The goal is to revolutionize the statistical prediction of turbulent fluid flows using a novel four-dimensional approach that is parallel in space as well as in time.

Navier-Stokes UPOs discovered to date were found using a “shooting” algorithm, whereby the fluid equations are integrated forward in time as the inner loop of a Newton-Raphson solver whose aim is to match the initial and final conditions. Researchers intend to extend these results by using a more efficient variational approach in space-time. The goal of this proposed work is the extensive cataloging of UPOs for chaotic and turbulent systems. This project aims to provide a greater understanding of the properties of turbulent flow, including a qualitatively new way to extract them from computation. This approach fully exploits and indeed could not be implemented without the compute, memory, and disk capabilities of leadership-class resources.

Results

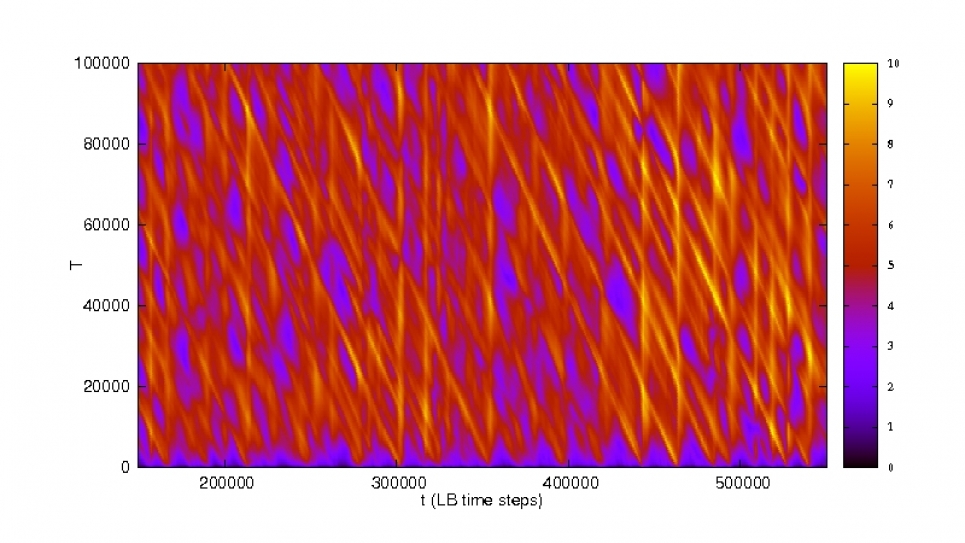
Using Intrepid, researchers have successfully validated their numerical procedures. They investigated different relaxation schemes, such as gradient descent and conjugate gradients, and have optimized parameters to facilitate convergence. It is believed that these are the first trials of a space-time minimization procedure with the goal of locating UPOs on such high-dimensional dynamical systems.

Improvements to the computational model will make vessel walls elastic. The result is a far more realistic model of how blood flows through the brain.

Future Efforts

Future efforts will focus on identification of UPOs of longer periods. In particular, researchers will collect a set of the shortest-period UPOs of driven homogeneous turbulence to test the dynamical zeta function approach to extracting turbulent averages.