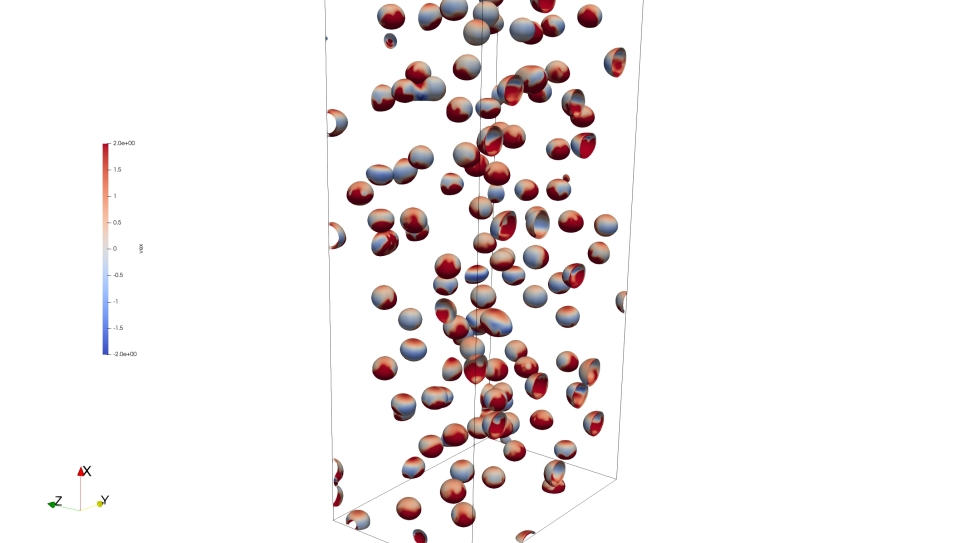
Transient vertical motion of bubbles due to buoyancy effects. Image Credit: Abbas Moradi Bilondi.
This team of researchers will carry out direct numerical simulations to study and quantify turbulence kinetic energy and diffusive scalar fields in gravity-driven turbulent bubbly suspensions.
Despite the wide range of applications involving bubbly flows, there is a lack of quantitative understanding regarding fluxes and mixing in turbulent flows. This understanding is crucial for accurate predictions and advancements in engineering systems/devices. This INCITE project aims to address this gap by conducting direct numerical simulations (DNS) to study and quantify turbulence kinetic energy and diffusive scalar fields in gravity-driven turbulent bubbly suspensions.
In various natural and practical applications, bubbly flows are integral to processes involving heat and mass transfer, as well as chemical reactions. However, a detailed quantitative comprehension of fluxes and mixing in these turbulent flows is essential for accurate predictions and technological progress in engineering. Thus, a key objective of this INCITE project is to employ fully-resolved direct numerical simulations (DNS) to investigate and measure the transport mechanisms of turbulence kinetic energy and a diffusive scalar field in gravity-driven turbulent bubbly suspensions.
This entails resolving all pertinent scales, including the bubble diameter, as well as the Kolmogorov and Bachelor scales, which correspond to the smallest vortices and scalar filaments in the flow, respectively. The team aims to facilitate high-performance computing (HPC) interface-resolved DNS of bubbly turbulent flows with passive scalars, focusing on scenarios with realistically low values of diffusivities.
This INCITE project outlines a systematic approach to uncover new theories and computational models for the dynamics of passive scalars in turbulence induced by bubbles. These complex interactions will be scrutinized through fully-resolved simulations. Unlike laboratory experiments, numerical studies allow for the isolation of specific physical processes. To minimize variations in bubble deformation resulting from changes in the Galilei number, the team will conduct simulations with varying surface tension. Consequently, if the bubble size increases (Galilei number), the surface tension will also increase, thereby maintaining a roughly consistent bubble deformation